Beyond the event horizon (4 photos)

Black holes exist. Although at first even scientists doubted the solution to their own equations. We were able to mathematically predict what the accretion disk should look like. In 2017, the Event Horizon Telescope photographed a black hole at the center of the M87 galaxy. She looks as expected. Mathematics is good at describing what is on this side of the event horizon, but what is hidden behind it?





Nothing! Having solved the equations, we get a singularity. But singularity is a mathematical term meaning the point at which a function tends to infinity. And physicists made from this an allegedly real physical object - a gravitational singularity - a point whose mass tends to infinity. But that doesn't make sense. A point has no spatial dimensions and cannot have mass.

It is impossible to prove that there is a singularity beyond the event horizon. Therefore, nothing prevents us from assuming that there is nothing beyond the horizon. Now I will try to explain how this is possible.

For easier understanding, let's consider two-dimensional space.

You can take a sheet of paper. Just remember that the paper has thickness, albeit small, and it is visible from the end. The plane will simply disappear from view, since its thickness is zero.

If we draw a circle in the center of a piece of paper and cut out the middle, we get a model of a black hole. A sheet is a two-dimensional plane. The cut line is an analogue of the event horizon - a one-dimensional object. A line only has length.



Where is the singularity in place of the missing piece of paper? And is it needed at all? Part of the plane has been removed. There is a boundary between the existing plane and its absence.

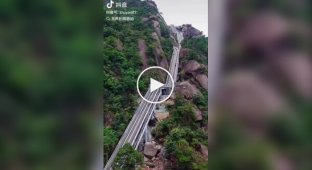
If we return to three-dimensional space, it may turn out that the event horizon is a lower-dimensional space, a two-dimensional surface that has no thickness. The border between the three-dimensional world and its absence. The edge of the universe is very close, in the center of the Milky Way, and not 46 billion light years away.

Nothing can leave the event horizon, not because gravity does not allow it, but because nothing can get beyond the event horizon. Once on it, the object becomes a flat image of itself. According to the theory of relativity, time will slow down more and more as it approaches the horizon until it stops. To an outside observer, objects never cross the event horizon.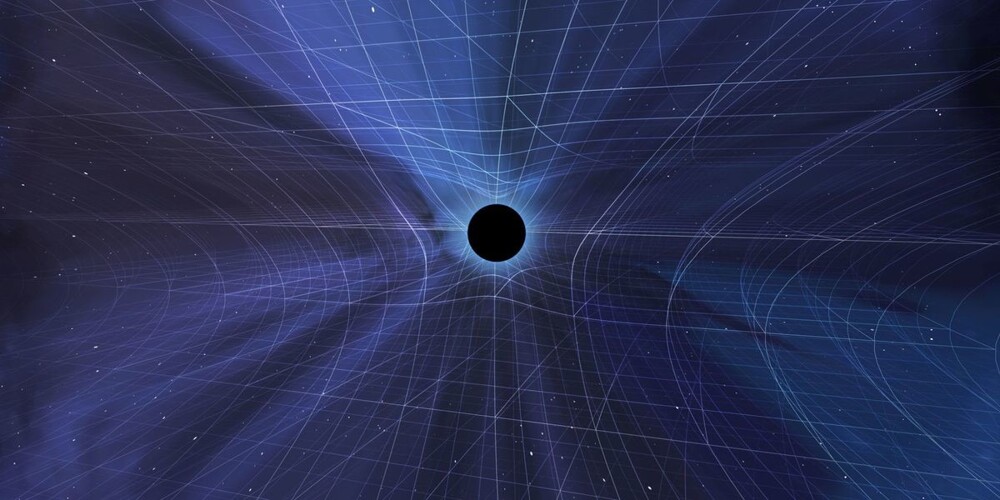





And since we're talking about gravity. Gravity is not a force. (Why they are trying to shove it into the theory of everything is unclear). Gravity is a consequence of the curvature of space. Until recently, scientists assumed that matter bends space. But then it suddenly turned out that 20 times more matter was needed to explain the observed curvatures, and they came up with Dark Matter. And since matter is equivalent to energy, it is also equivalent to Dark Energy. Neither one nor the other is needed if we assume that the space around us is simply “curved” in itself. And matter collects in certain places due to the geometry of space itself.

A black hole is just such a feature in the geometry of space. A location of dimensional change around which a "tension" occurs, measured as gravity. Matter is not needed for this, but it accumulates around, forming galaxies and quasars.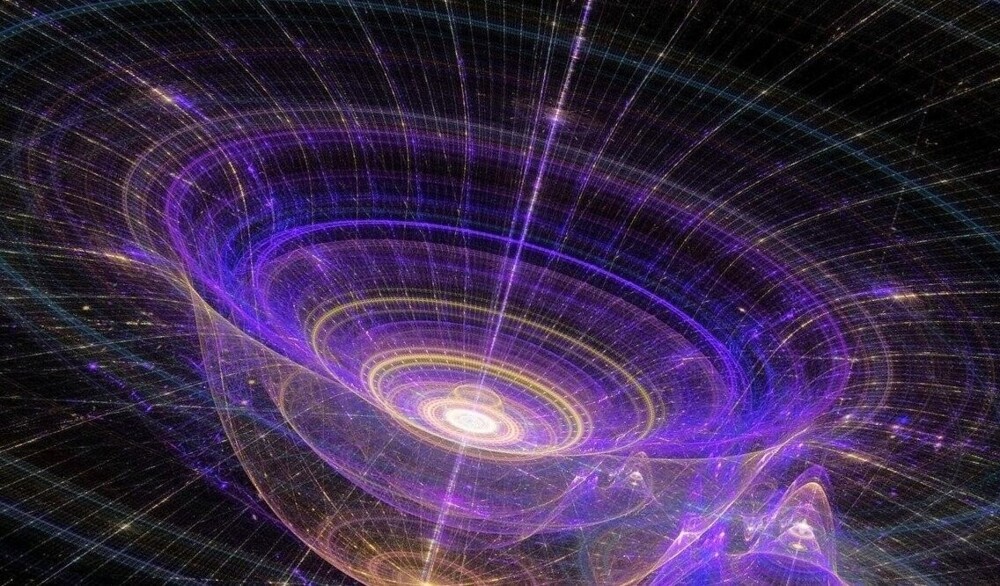



Summary.

The event horizon is a two-dimensional plane closed on itself in the shape of a sphere. Any three-dimensional object falling on this plane becomes an image of the object. The image cannot leave the plane and become an object, so it is impossible to leave the event horizon. All information about the object is preserved in the image (holographic principle)

Beyond the event horizon there is no space and time, no matter. Mass is a measure of the curvature of space. It makes no difference whether space is curved by matter or without it, the same curvature will show the same measurement of mass.

P.S. While I was writing the article, I remembered the books by Sergei Snegov “People Like Gods” and Liu Cixin’s “The Three-Body Problem”. Both are very good science fiction. And in both there are interesting views of the authors on the geometry and properties of space. I recommend reading it.